

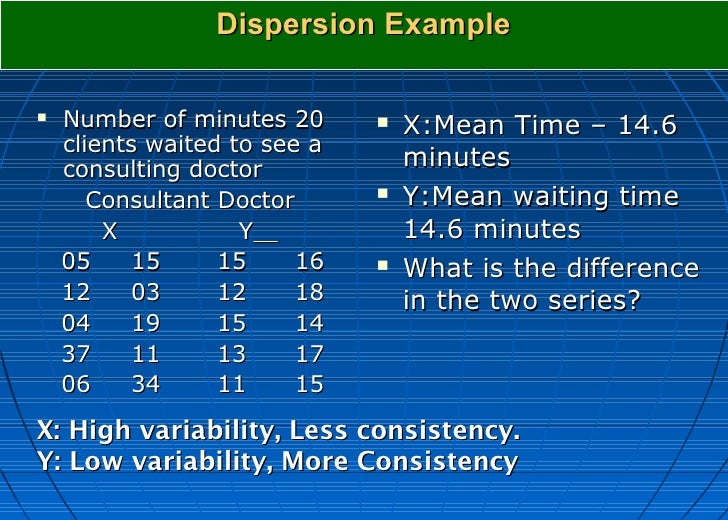
Range: We calculate it by subtracting the smallest score from the largest score in the data set. The range is the simplest measure of variability and is really easy to calculate. Exam scores for 2 sections of a class.Ĭomparing both sections you can see that the scores for Section A very few scores are represented (e.g., 70 and 85) and they are very far from the mean, while in Section B more scores are represented and clustered close to the mean We would say that the spread of scores for Section A is greater than Section B. Let’s examine the scores for each section. If that is the only descriptive statistic I report you might assume that both classes are identical – but that is not necessarily true. Imagine that students in two different sections of statistics take Exam 1 and the mean score in both classrooms is a 75. An important characteristic of any set of data is the variation in the data. In this chapter, we will look at three measures of variability: range, variance, and standard deviation. Examples of 2 normal (symmetrical, unimodal) distributions. For the “small” distribution in Figure 1, the data values are concentrated closely near the mean in the “large” distribution, the data values are more widely spread out from the mean.įigure 1. The taller-looking distribution shows a smaller dispersion while the wider distribution shows a larger dispersion. What is different between the two is the spread or dispersion of the scores. You can see both are normally distributed (unimodal, symmetrical), and the mean, median, and mode for both fall on the same point. There are two distributions, labeled as small and large. In Figure 1, the y-axis is frequency and the x-axis represents values for a variable.
The more spread out the scores are, the higher the dispersion or spread. Measures of dispersion describe the spread of scores in a distribution. Just as in the section on central tendency where we discussed measures of the center of a distribution of scores, in this chapter we will discuss measures of the variability of a distribution. The terms variability, spread, and dispersion are synonyms, and refer to how spread out a distribution is. Measure of central tendency (a value around which other scores in the set cluster) and a measure of variability (an indicator of how spread out about the mean scores are in a data set) are used together to give a description of the data.


 0 kommentar(er)
0 kommentar(er)
